In this lab, you will learn that some ways to solve a problem are faster than others.
On this page, you will compare two algorithms for adding the numbers from 1 to an input number (as shown below).

We should change the CSS so that sidenotes never extend behind the element they are about; add space to the element if necessary. For example, that "Read this out loud" box shouldn't overlap the orange. --MF, 12/27/17
Brian also wants to change the katex in 5 and make the narrower box light blue here. --MF, 12/27/17
Fundamental concern: this context is too much overhead for teaching the idea of efficiency; the context should be more accessible so efficiency is the only idea they are grappling with. --MF, 9/26/18
PG: I've never loved any part of this lab, but we need the content in some form. This page takes the special case of triangular numbers and shows how the closed form is clearly more efficient than anything else: the positive message is that a bit of forethought (mathematics) can be efficient. The problem is that it is such a special case and that combine gets dismissed so quickly. I've also never been thrilled with the timer.
BH: This whole unit needs rethinking. Limiting the discussion to what the AP requires makes it boring. Bring back activities about all the categories from constant to exponential time, including n log n, etc.
The numbers from A to B block is now in the tools library.
MF: needs formatting edits, but more importantly, the page is too long and the context is too much overhead to teach efficiency
In this lab, you will learn that some ways to solve a problem are faster than others.
On this page, you will compare two algorithms for adding the numbers from 1 to an input number (as shown below).


combine to add up the list.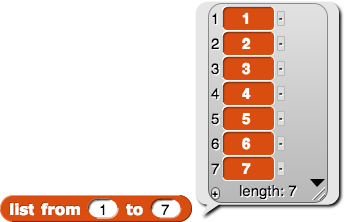

| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 |
| 13 | 12 | 11 | 10 | 9 | 8 | 7 | 6 | 5 | 4 | 3 | 2 | 1 |
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | |
| + | 13 | 12 | 11 | 10 | 9 | 8 | 7 | 6 | 5 | 4 | 3 | 2 | 1 |
| 14 | 14 | 14 | 14 | 14 | 14 | 14 | 14 | 14 | 14 | 14 | 14 | 14 |
numbers from () to () in the Variables palette. You'll need to sum from 1 to algorithm. You can use Morgan’s method (using combine to code sum), you can use Jasmine’s formula, or you can build it your own way.sum from 1 to( x).